Time limit: 0
Finish Test
0 of 18 questions completed
Questions:
- 1
- 2
- 3
- 4
- 5
- 6
- 7
- 8
- 9
- 10
- 11
- 12
- 13
- 14
- 15
- 16
- 17
- 18
Information
- Most Important Multiple Choice Questions
- Online Pipes and Cisterns Exercise with Correct Answer Key and Solutions
- Useful for all Competitive Exams
You have already completed the quiz before. Hence you can not start it again.
Test is loading...
You must sign in or sign up to start the quiz.
You have to finish following quiz, to start this quiz:
Results
0 of 18 questions answered correctly
Time has elapsed
You have reached 0 of 0 points, (0)
Categories
- Not categorized 0%
- 1
- 2
- 3
- 4
- 5
- 6
- 7
- 8
- 9
- 10
- 11
- 12
- 13
- 14
- 15
- 16
- 17
- 18
- Answered
- Review
- Question 1 of 18
1. Question
Two taps can fill a tank in 15 and 12 min, respectively. A third tap can empty it in 20 min. If all the taps are opened at the same time, then in how much time will the tank be filled?
Hint
Part of tank filled in one minute in given condition
∴ Tank will be completely filled in 10 min.
- Question 2 of 18
2. Question
Two taps can fill a tank in 20 minutes and 30 minutes respectively. There is an outlet tap at exactly half level of that rectangular tank which can pump out 50 litres of water per minute. If the outlet tap is open, then it takes 24 minutes to fill an empty tank. What is the volume of the tank?
Hint
The two filler tap can fill the
part of tank in 1 min.∴ The two filler tap can fill the tank in 12 min.
∴ Half of the tank will be filled in 6 min.
Hence, it took (24 – 6 = 18 min.) to fill the remaining half of the tank when the outlet pump is opened.
Thus, the total time required to empty half of the tank
minutesThus, capacity of the tank
= 100 × 9 × 2
= 1800 litres
- Question 3 of 18
3. Question
Two taps can separately fill a cistern in 10 minutes and 15 minutes, respectively and when the waste pipe is open, they can together fill it in 18 minutes. The waste pipe can empty the full cistern in :
Hint
Time taken by one tap to fill the cistern = ¹⁄₁₀ hr
and second tap fills the cistern = ¹⁄₁₅ hr
The time taken by the both tap to fill the cistern
=
=
Thus, both tap fill the cistern in 6 minutes.
Now, given when waste pipe is open, both can fill the cistern in ¹⁄₁₈ hr
Time taken by waste pipe to empty the cistern
=
hrs=
= ¹⁄₉ minutes
Hence, in 9 minutes waste pipe can empty the cistern.
- Question 4 of 18
4. Question
One fill pipe A is 3 times faster than second fill pipe B and takes 10 minutes less time to fill a cistern than B takes. Find when the cistern will be full if fill pipe B is only opened.
Hint
Let B can fill the cistern in x min. Then,
then A can fill the cistern in
Given
- Question 5 of 18
5. Question
Two pipes A and B can fill a tank in 15 and 12 hours respectively. Pipe B alone is kept open for ¾ of time and both pipes are kept open for remaining time. In how many hours, the tank will be full?
Hint
Let the required time be x hours, then
⇒
⇒ x = 10 hours
- Question 6 of 18
6. Question
A water tank has three taps A, B and C. A fills four buckets in 24 minutes, B fills 8 buckets in 1 hour and C fills 2 buckets in 20 minutes. If all the taps are opened together a full tank is emptied in 2 hours. If a bucket can hold 5 litres of water, what is the capacity of the tank?
Hint
Tap A fills 4 buckets (4 × 5 = 20 litres) in 24 min.
In 1 hour tap A fills
In 1 hour tap B fills = 8 × 5 = 40
In 1 hour tap C fills
If they open together they would fill
50 + 40 + 30 = 120 litres in one hour
but full tank is emptied in 2 hours
So, tank capacity would be 120 × 2 = 240 litres.
- Question 7 of 18
7. Question
A pump can be operated both for filling a tank and for emptying it. The capacity of tank is 2400 m³. The emptying capacity of the pump is 10 m³ per minute higher than its filling capacity. Consequently, the pump needs 8 minutes less to empty the tank to fill it. Find the filling capacity of pump.
Hint
Let the filling capacity of pump be x m³/min.
Then, emptying capacity of pump
= (x + 10) m³ /min.
∴
⇒ x² + 10 x – 3000 = 0
⇒ (x – 50)(x + 60) = 0
⇒ x = 50 m³/min.
- Question 8 of 18
8. Question
Filling pipe, if opened alone, takes 5 minutes to fill a cistern. Suddenly, during the course of fillling, the waste pipe (which is of similar size and flow as of fill pipe) is opened for 2 minutes, then the cistern will be filled in
Hint
Since, flow of waste pipe = flow of filling pipe.
⇒ Filled part in one min = emptied part in one min.
∴ After opening the waste pipe for 2 min, cistern will be full in
(5 + 2) = 7 min.
- Question 9 of 18
9. Question
A cistern has three pipes, A, B and C. The pipes A and B can fill it in 4 and 5 hours respectively and C can empty it in 2 hours. If the pipes are opened in order at 1, 2 and 3 a.m. respectively, when will the cistern be empty?
Hint
Let the time be t hours after 1 a.m.
∴
- Question 10 of 18
10. Question
A pipe can fill a cistern in 6 hours. Due to a leak in its bottom, it is filled in 7 hours. When the cistern is full, in how much time will it be emptied by the leak?
Hint
Part of the capacity of the cistern emptied by the leak in one hour
=
of the cistern.The whole cistern will be emptied in 42 hours.
- Question 11 of 18
11. Question
A tank is normally filled in 8 hrs but takes 2 hrs longer to fill because of a leak in its bottom. If the cistern is full, in how many hrs will the leak empty it?
Hint
It is clear from the question that the filler pipe fills the tank in 8 hrs and if both the filler and the leak work together, the tank is filled in 8 hrs .
Therefore the leak will empty the tank in
= 40 hrs. - Question 12 of 18
12. Question
An electric pump can fill a tank in 3 hours. Because of a leak in the tank it was taking 3.5 hours to fill the tank. Find the time in which the leak can drain all the water of the tank when full.
Hint
Part of tank filled in 1 hour = 1/3
Part of tank emptied in the same time
=
Total time required to empty it
=
- Question 13 of 18
13. Question
Two pipes A and B can fill a tank in 20 and 30 hours respectively. Both the pipes are opened to fill the tank but when the tank is 1/3rd full, a leak develops in the tank through which one-third water supplied by both the pipes goes out. The total time taken to fill the tank is
Hint
The pipes A and B together can fill
of the tank in one hour.∴ ⅓ of the tank is filled by both the pipes A and B together in 4 hours. …(1)
Now because of developing a leak after 4 hours, both the pipes can fill
of the tank in one hour[ Because ⅓rd of the water supplied by both the pipes goes out]
∴ Remaining ⅔ of the tank can be filled by both the pipes in
⅔ × 18 = 12 hours … (2)
∴ The total time taken to fill the tank is 16 hours.
- Question 14 of 18
14. Question
Three pipes A, B and C can fill a tank from empty to full in 30 minutes, 20 minutes and 10 minutes respectively. When the tank is empty, all the three pipes are opened. A , B and C discharge chemical solutions P, Q and R respectively. What is the proportion of solution R in the liquid in the tank after 3 minutes?
Hint
Part filled by (A + B + C) in 3 minutes
=
Part filled by C in 3 minutes
=
∴ Required ratio
=
- Question 15 of 18
15. Question
A cistern can be filled by two pipes filling separately in 12 and 16 min. respectively. Both pipes are opened together for a certain time but being clogged, only 7/8 of the full quantity of water flows through the former and only 5/6 through the latter pipe. The obstructions, however, being suddenly removed, the cistern is filled in 3 min. from that moment. How long was it before the full flow began?
Hint
Both the pipes A and B can fill
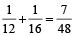of the cistern in one minute, when their is no obstruction.With obstruction, both the pipes can fill
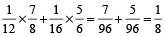of the cistern in one minute.Let the obstructions were suddenly removed after x minutes.
∴ With obstruction, x/8 of the cistern could be filled in x minutes and so the remaining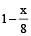
of the cistern was filled without obstruction in 3 minutes,i.e. In one minute,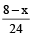
of the cistern was filled with obstruction.
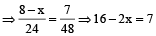
⇒ x = 4.5 min.
- Question 16 of 18
16. Question
A volcanic crater (conical) has a base diameter 125 m and is 10 m deep. It rains very heavily and the crater gets filled up in 4 hours. Find the rate of water flow in the crater.
Hint
Volume of the cone
=
⇒ Rate of water flow
=
- Question 17 of 18
17. Question
Water flows at 3 metres per sec through a pipe of radius 4 cm. How many hours will it take to fill a tank 40 metres long, 30 metres broad and 8 metres deep, if the pipe remains full?
Hint
Radius of the pipe (r)
= 4 cm. = 0.04 meter
Volume of water flowing out per sec
= πr² × rate of flow
=
cubic metres= 0.0151 cubic metres
Time taken to fill the tank
= 40 × 30 ×
sec=
- Question 18 of 18
18. Question
An outlet pipe empties a tank which is full, in 10 hours. If the inlet pipe is kept open, which lets water in at the rate of 8 litres/minute, the outlet pipe would take 6 hours longer. Find the capacity of the tank.
Hint
Part filled by the inlet pipe in 1 hour
=
= 
= 
Part filled by the inlet pipe in 1 minute
=
= 
∴ Capacity of tank
= 1600 × 8 = 12800 litres.